Skip to main content
SpringerLink
Log in

Explicit invariant solutions associated with nonlinear atmospheric flows in a thin rotating spherical shell with and without west-to-east jets perturbations

  • Published:
Analysis and Mathematical Physics Aims and scope Submit manuscript

Abstract

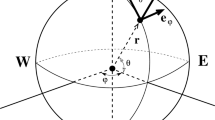
A class of non-stationary exact solutions of two-dimensional nonlinear Navier–Stokes (NS) equations within a thin rotating spherical shell were found as invariant and approximately invariant solutions. The model is used to describe a simple zonally averaged atmospheric circulation caused by the difference in temperature between the equator and the poles. Coriolis effects are generated by pseudoforces, which support the stable west-to-east flows providing the achievable meteorological flows. The model is superimposed by a stationary latitude dependent flow. Under the assumption of no friction, the perturbed model describes zonal west-to-east flows in the upper atmosphere between the Ferrel and Polar cells. In terms of nonlinear modeling for the NS equations, two small parameters are chosen for the viscosity and the rate of the earth’s rotation and exact solutions in terms of elementary functions are found using approximate symmetry analysis. It is shown that approximately invariant solutions are also valid in the absence of the flow perturbation to a zonally averaged mean flow.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3

Similar content being viewed by others

Notes

  1. We use here the term “exact” when referring to the classical Lie point symmetries of a given system. This is in order to make the distinction clear between such symmetries and their approximate symmetry counterparts.

References

  1. Anderson, R.F., Ali, S., Brandtmiller, L.L., Nielsen, S.H.H., Fleisher, M.Q.: Wind-driven upwelling in the Southern Ocean and the deglacial rise in atmosheric \(CO_{2}\). Science 323, 1443–1448 (2006)

    Article  Google Scholar 

  2. Baikov, V.A., Gazizov, R.K., Ibragimov, N.H., 1988: Approximate symmetries of equations with a small parameter, Mat. Sb. 136, 435–450 (English Transl. in Math. USSR Sb. 64 (1989), 427–441)

  3. Bachelor, G.K.: An Introduction to Fluid Dynamics. Cambridge University Press, Cambridge (1967)

    Google Scholar 

  4. Balasuriya, S.: Vanishing viscosity in the barotropic \( \beta -\)plane. J. Math. Anal. Appl. 214, 128–150 (1997)

    Article  MathSciNet  MATH  Google Scholar 

  5. Belotserkovskii, O.M., Mingalev, I.V., Mingalev, O.V.: Formation of large-scale vortices in shear flows of the lower atmosphere of the earth in the region of tropical latitudes. Cosm. Res. 47(6), 466–479 (2009)

    Article  Google Scholar 

  6. Ben-Yu, G.: Spectral method for vorticity equations on spherical surface. Math. Comput. 64, 1067–1079 (1995)

    Article  Google Scholar 

  7. Blinova, E.N.: A hydrodynamical theory of pressure and temperature waves and of centres of atmospheric action, C.R. (Doklady) Acad. Sci USSR 39, 257–260 (1943)

    MathSciNet  MATH  Google Scholar 

  8. Blinova, E.N.: A method of solution of the nonlinear problem of atmospheric motions on a planetary scale. Dokl. Acad. Nauk USSR 110, 975–977 (1956)

    MathSciNet  MATH  Google Scholar 

  9. Caflisch, R.E., Siegel, M.: A semi-analytical approach to Euler singularties. Methos. Appl. Anal. 11(3), 001–007 (2004)

    MathSciNet  Google Scholar 

  10. Callaghan, T.G., Forbes, L.K.: Computing large-scale progressive Rossby waves on a sphere. J. Comput. Phys. 217, 845 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  11. Cenedese, C., Linden, P.F.: Cyclone and anticyclone formation in a rotating stratified fluid over a sloping bottom. J. Fluid Mech. 381, 199–223 (1999)

    Article  MathSciNet  MATH  Google Scholar 

  12. Fushchych, W.I., Shtelen, W.H.: On approximate symmetry and approximate solution of the non-linear wave equation with a small parameter. J. Phys. A: Math. Gen. 22, 887–890 (1989)

    Article  Google Scholar 

  13. Golovkin, H.: Vanishing viscosity in Cauchy’s problem for hydromechanics equation. Proc. Steklov Inst. Math. 92, 33–53 (1966)

    Google Scholar 

  14. Herrmann, E.: The motions of the atmosphere and especially its waves. Bull. Amer. Math. Soc. 2(9), 285–296 (1896)

    Article  MathSciNet  Google Scholar 

  15. Ibragimov, R.N.: Shallow water theory and solutions of the free boundary problem on the atmospheric motion around the Earth. Physica Scripta 61, 391–395 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  16. Ibragimov, R.N.: Approximate equivalence transformations and invariant solutions of a perturbed nonlinear wave equation. Electron. J. Differ. Equ. 23, 1–12 (2001)

    Google Scholar 

  17. Ibragimov, R.N.: Generation of internal tides by an oscillating background flow along a corrugated slope. Physica Scripta 78, 065801 (2008)

    Article  Google Scholar 

  18. Ibragimov, R.N.: Effects of rotation on self-resonant internal gravity waves in the ocean. Ocean Model 31, 80–87 (2010)

    Article  Google Scholar 

  19. Ibragimov, R.N.: Nonlinear viscous fluid patterns in a thin rotating spherical domain and applications. Phys. Fluids 23, 123102 (2011)

    Article  Google Scholar 

  20. Ibragimov, R.N., Dameron, M.: Spinning phenomena and energetics of spherically pulsating patterns in stratified fluids. Physica Scripta 84, 015402 (2011)

    Article  Google Scholar 

  21. Ibragimov, R.N., Pelinovsky, D.E.: Incompressible viscous fluid flows in a thin spherical shell. J. Math. Fluid. Mech. 11, 60–90 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  22. Ibragimov, R.N., Pelinovsky, D.E.: Effects of rotation on stability of viscous stationary flows on a spherical surface. Phys. Fluids 22, 126602 (2010)

    Article  Google Scholar 

  23. Ibragimov, R.N., Yilmaz, N.: Experimental mixing parameterization due to multiphase fluid-structure interactions. Mech. Res. Commun. 38, 261–266 (2011)

    Article  Google Scholar 

  24. Ibragimov, N.H., Ibragimov, R.N.: Integration by quadratures of the nonlinear Euler equations modeling atmospheric flows in a thin rotating spherical shell. Phys. Lett. A. 375, 3858–3865 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  25. Ibragimov, N.H., Ibragimov, R.N.: Applications of Lie Group Analysis in Geophysical Fluid Dynamics, Series of Complexity, Nonlinearity and Chaos, vol. 2. World Scientific Publishers (2011)

  26. Ibragimov, R.N., Jefferson, G., Carminati, J.: Invariant and approximately invariant solutions of non-linear internal gravity waves forming a column of stratified fluid affected by the Earth’s rotation. Int J. Non-Linear Mech. 51, 28–44 (2013)

    Article  Google Scholar 

  27. Ibragimov, N.H.: Elementary Lie Group Analysis and Ordinary Differential Equations. John Wiley & Sons, Chichester (1999)

    MATH  Google Scholar 

  28. Iftimie, D., Raugel, G.: Some results on the Navier-Stokes equations in thin 3D domains. J. Diff. Eqs. 169, 281–331 (2001)

    Article  MathSciNet  MATH  Google Scholar 

  29. Jefferson, G.F., Carminati, J., 2011: ASP: Approximate Symbolic Computation of Approximate Symmetries of Differential Equations, Computer Physics Communications, submitted 2012.

  30. Lamb, H.: Hydrodynamics, 5 5 edn. Cambridge University Press, New York (1924)

    MATH  Google Scholar 

  31. Lions, J.L., Teman, R., Wang, S.: On the equations of the large-scale ocean. Nonlinearity 5, 1007–1053 (1992)

    Article  MathSciNet  MATH  Google Scholar 

  32. Lions, J.L., Teman, R., Wang, S.: New formulations of the primitive equations of atmosphere and applications. Nonlinearity 5, 237–288 (1992)

    Article  MathSciNet  MATH  Google Scholar 

  33. Ohkitani, K., Gibbon, J.D.: Numerical study of singularity formation in a class of Euler and Navier-Stokes flows. Phys. Fluids 12, 3181–3194 (2000)

    Article  MathSciNet  Google Scholar 

  34. Pakdemirli, M., Yürüsoy, M., Dolapçi, İ.T.: Comparison of approximate symmetry methods for differential equations. Acta Applicandae Mathematicae 80, 243–271 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  35. Shindell, D.T., Schmidt, G.A.: Southern Hemisphere climate response to ozone changes and greenhouse gas increases. Res. Lett. 31, L18209 (2004)

    Article  Google Scholar 

  36. Sleijpen, G.L.G., Van der Vorst, H.A.: A Jacobi-Davidson iteration method for linear eigenvalue problems. SIAM J. Matrix Anal. Appl. 17, 410–425 (1996)

    Article  Google Scholar 

  37. Shen, J.: 1992: On pressure stabilization method and projection method for unsteady Navier-Stokes equations. Advances in Computer Methods for Partial Differential Equations, pp. 658–662. IMACS, New Brunswick, NJ (1992)

  38. Summerhayes, C.P., Thorpe, S.A.: Oceanography. John Willey & Sons, An Illustrative Guide, New York (1996)

  39. Swarztrauber, P.N.: Shallow water flow on the sphere. Mon. Weather Rev. 132, 3010 (2004)

    Article  Google Scholar 

  40. Temam, R., Ziane, M.: Navier-Stokes equations in thin spherical domains. Contemp. Math. 209, 281–314 (1997)

    Article  MathSciNet  Google Scholar 

  41. Toggweiler, J.R., Russel, J.L.: Ocean circulation on a warming climate. Nature. 451, 286–288 (2008)

    Article  Google Scholar 

  42. Vallis, G.K.: Atmospheric anf Ocean Fluid Dynamics. Cambridge University Pres, New York (2006)

    Book  Google Scholar 

  43. Vu, K.T., Jefferson, G.F., Carminati, J.: Finding generalised symmetries of differential equations using the MAPLE package DESOLVII. Comput. Phys. Commun. 183, 1044–1054 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  44. Zeidler, E.: Applied Functional Analysis: Applications to Mathematical Physics. Springer-Verlag, New York (1995)

    Google Scholar 

  45. Zeytounian, R.Kh., Guiraud, J.P., 1980: Acad. Sci., Paris 290 B, 75.

  46. Weijer, W., Vivier, F., Gille, S.T., Dijkstra, H.: Multiple oscillatory modes of the Argentine Basin. Part II: The spectral origin of basin modes. J. Phys. Oceanogr. 37, 2869–2881 (2007)

    Article  Google Scholar 

  47. Williamson, D.: A standard test for numerical approximation to the shallow water equations in spherical geometry. J. Comput. Phys. 102, 211–224 (1992)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Acknowledgments

This research was supported in part by an appointment to the U.S. Department of Energy’s Visiting Faculty Program.

Author information

Authors and Affiliations

  1. Pacific Northwest National Laboratory, Richland, WA , 99352, USA

    Ranis Ibragimov

  2. School of Information Technology, Deakin University, Waurn Ponds, Australia

    Grace Jefferson & John Carminati

Authors
  1. Ranis Ibragimov
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Grace Jefferson
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. John Carminati
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Ranis Ibragimov.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Ibragimov, R., Jefferson, G. & Carminati, J. Explicit invariant solutions associated with nonlinear atmospheric flows in a thin rotating spherical shell with and without west-to-east jets perturbations. Anal.Math.Phys. 3, 375–391 (2013). https://doi.org/10.1007/s13324-013-0062-9

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s13324-013-0062-9

Keywords

Search

Navigation