Skip to main content
SpringerLink
Log in

Dimension-Free Entanglement Detection in Multipartite Werner States

  • Published:
Communications in Mathematical Physics Aims and scope Submit manuscript

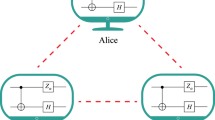
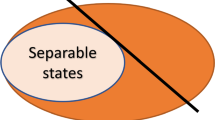
Abstract

Werner states are multipartite quantum states that are invariant under the diagonal conjugate action of the unitary group. This paper gives a complete characterization of their entanglement that is independent of the underlying local Hilbert space: for every entangled Werner state there exists a dimension-free entanglement witness. The construction of such a witness is formulated as an optimization problem. To solve it, two semidefinite programming hierarchies are introduced. The first one is derived using real algebraic geometry applied to positive polynomials in the entries of a Gram matrix, and is complete in the sense that for every entangled Werner state it converges to a witness. The second one is based on a sum-of-squares certificate for the positivity of trace polynomials in noncommuting variables, and is a relaxation that involves smaller semidefinite constraints.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Affleck, I., Kennedy, T., Lieb, E.H., Tasaki, H.: Rigorous results on valence-bond ground states in antiferromagnets. Phys. Rev. Lett. 59, 799–802 (1987)

    Article  ADS  Google Scholar 

  2. Anjos, M.F., Lasserre, J.B. (eds.): Handbook on semidefinite, conic and polynomial optimization. In: International Series in Operations Research and Management Science, vol. 166. Springer, New York (2012)

  3. Berta, M., Borderi, F., Fawzi, O., Scholz, V.B.: Semidefinite programming hierarchies for constrained bilinear optimization. Math. Program. 194, 781–829 (2022)

    Article  MathSciNet  MATH  Google Scholar 

  4. Blekherman, G., Parrilo, P.A., Thomas, R.R. (eds.): Semidefinite Optimization and Convex Algebraic Geometry, Society for Industrial and Applied Mathematics (2013)

  5. Brandao, F.G., Vianna, R.O.: Robust semidefinite programming approach to the separability problem. Phys. Rev. A 70, 062309 (2004)

    Article  ADS  Google Scholar 

  6. Bohnet-Waldraff, F., Braun, D., Giraud, O.: Entanglement and the truncated moment problem. Phys. Rev. A 96, 032312 (2017)

    Article  ADS  Google Scholar 

  7. Christandl, M., König, R., Mitchison, G., Renner, R.: One-and-a-half quantum de Finetti theorems. Commun. Math. Phys. 273, 473–498 (2007)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  8. Cafuta, K., Klep, I., Povh, J.: Rational sums of Hermitian squares of free noncommutative polynomials. Ars Math. Contemp. 9(2), 243–259 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  9. Collins, B., Śniady, P.: Integration with respect to the Haar measure on unitary, orthogonal and symplectic group. Commun. Math. Phys. 264, 773–795 (2006)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  10. Cavalcanti, D., Skrzypczyk, P.: Quantum steering: a review with focus on semidefinite programming. Rep. Progr. Phys. 80(2), 024001 (2016)

    Article  ADS  MathSciNet  Google Scholar 

  11. Doherty, A.C., Parrilo, P.A., Spedalieri, F.M.: Complete family of separability criteria. Phys. Rev. A 69, 022308 (2004)

    Article  ADS  Google Scholar 

  12. Eisert, J., Hyllus, P., Gühne, O., Curty, M.: Complete hierarchies of efficient approximations to problems in entanglement theory. Phys. Rev. A 70, 062317 (2004)

    Article  ADS  Google Scholar 

  13. Eggeling, T., Werner, R.F.: Separability properties of tripartite states with \(U \otimes U \otimes U\) symmetry. Phys. Rev. A 63, 042111 (2001)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  14. Frérot, I., Baccari, F., Acín, A.: Unveiling quantum entanglement in many-body systems from partial information. PRX Quantum 3, 010342 (2022)

    Article  ADS  Google Scholar 

  15. Fulton, W., Harris, J.: Representation Theory: A First Course, Graduate Texts in Mathematics, vol. 129. Springer, New York (2004)

    Google Scholar 

  16. Grone, R., Merris, R., Watkins, W.: Cones in the group algebra related to Schur’s determinantal inequality. Rocky Mt. J. Math. 18(1), 137–146 (1988)

    Article  MathSciNet  MATH  Google Scholar 

  17. Gühne, O., Tóth, G.: Entanglement detection. Phys. Rep. 474(1), 1 (2009)

    Article  ADS  MathSciNet  Google Scholar 

  18. Gurvits, L.: Classical deterministic complexity of Edmond’s problem and quantum entanglement. In: Proceedings of the Thirty-Fifth Annual ACM Symposium on Theory of Computing, ACM, New York, pp. 10–19 (2003)

  19. Horodecki, M., Horodecki, P., Horodecki, R.: Separability of mixed states: necessary and sufficient conditions. Phys. Lett. A 223(1), 1 (1996)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  20. Huber, F., Maassen, H.: Matrix forms of immanant inequalities (preprint) (2021). https://arxiv.org/abs/2103.04317

  21. Harrow, A.W., Natarajan, A., Xiaodi, W.: An improved semidefinite programming hierarchy for testing entanglement. Commun. Math. Phys. 352, 881–904 (2017)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  22. Huber, F.: Positive maps and trace polynomials from the symmetric group. J. Math. Phys. 62(2), 022203 (2021)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  23. Jungnitsch, B., Moroder, T., Gühne, O.: Taming multiparticle entanglement. Phys. Rev. Lett. 106, 190502 (2011)

    Article  ADS  Google Scholar 

  24. Ji, Z., Natarajan, A., Vidick, T., Wright, J., Yuen, H.: MIP*=RE. Commun. ACM 64, 131–138 (2021). arXiv:2001.04383

  25. Klep, I., Magron, V., Povh, J.: Sparse noncommutative polynomial optimization. Math. Program. 193, 789–829 (2022)

    Article  MathSciNet  MATH  Google Scholar 

  26. Klep, I., Magron, V., Volčič, J.: Optimization over trace polynomials. Ann. Henri Poincaré 23, 67–100 (2022)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  27. Kostant, B.: A theorem of Frobenius, a theorem of Amitsur–Levitski and cohomology theory. J. Math. Mech. 7, 237–264 (1958)

    MathSciNet  MATH  Google Scholar 

  28. Lasserre, J.B.: Global optimization with polynomials and the problem of moments. SIAM J. Optim. 11(3), 796–817 (2000/01)

  29. Lancien, C., Gühne, O., Sengupta, R., Huber, M.: Relaxations of separability in multipartite systems: semidefinite programs, witnesses and volumes. J. Phys. A Math. Theor. 48(50), 505302 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  30. Mironowicz, P.: Applications of semi-definite optimization in quantum information protocols. PhD Thesis, Gdańsk University of Technology (2018). https://arxiv.org/abs/1810.05145

  31. Maassen, H., Kümmerer, B.: Entanglement of symmetric Werner states. http://www.bjadres.nl/MathQuantWorkshop/Slides/SymmWernerHandout.pdf (2019)

  32. Marcus, M., Minc, H.: Generalized matrix functions. Trans. Am. Math. Soc. 116, 316–329 (1965)

    Article  MathSciNet  MATH  Google Scholar 

  33. Mozrzymas, M., Studziński, M., Horodecki, M.: A simplified formalism of the algebra of partially transposed permutation operators with applications. J. Phys. A 51(12), 125202 (2018)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  34. Navascués, M., Owari, M., Plenio, M.B.: Power of symmetric extensions for entanglement detection. Phys. Rev. A 80, 052306 (2009)

    Article  ADS  MATH  Google Scholar 

  35. Navascués, M., Pironio, S., Acín, A.: A convergent hierarchy of semidefinite programs characterizing the set of quantum correlations. New J. Phys. 10(7), 073013 (2008)

    Article  ADS  Google Scholar 

  36. Peres, A.: Separability criterion for density matrices. Phys. Rev. Lett. 77, 1413–1415 (1996)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  37. Peyrl, H., Parrilo, P.A.: Computing sum of squares decompositions with rational coefficients. Theor. Comput. Sci. 409(2), 269–281 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  38. Procesi, C.: Lie Groups. An Approach through Invariants and Representations. Springer, New York (2007)

  39. Procesi, C.: A note on the Formanek Weingarten function. Note Mat. 41, 69–109 (2021)

    MathSciNet  MATH  Google Scholar 

  40. Putinar, M.: Positive polynomials on compact semi-algebraic sets. Indiana Univ. Math. J. 42(3), 969–984 (1993)

    Article  MathSciNet  MATH  Google Scholar 

  41. Riener, C., Theobald, T., Andrén, L.J., Lasserre, J.B.: Exploiting symmetries in sdp-relaxations for polynomial optimization. Math. Oper. Res. 38(1), 122–141 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  42. Stasińska, J., Rogers, B., Paternostro, M., De Chiara, G., Sanpera, A.: Long-range multipartite entanglement close to a first-order quantum phase transition. Phys. Rev. A 89, 032330 (2014)

    Article  ADS  Google Scholar 

  43. Scherer, C.W., Hol, C.W.J.: Matrix sum-of-squares relaxations for robust semi-definite programs. Math. Program. 107, 189–211 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  44. Studziński, M., Mozrzymas, M., Kopszak, P., Horodecki, M.: Efficient multi port-based teleportation schemes. IEEE Trans. Inf. Theory (2022)

  45. Vandenberghe, L., Boyd, S.: Semidefinite programming. SIAM Rev. 38(1), 49 (1996)

    Article  MathSciNet  MATH  Google Scholar 

  46. Vianna, R.O., Doherty, A.C.: Distillability of Werner states using entanglement witnesses and robust semidefinite programs. Phys. Rev. A 74, 052306 (2006)

    Article  ADS  Google Scholar 

  47. Vinzant, C.: What is a spectrahedron? AMS Notices 61(5), 492 (2014)

    MathSciNet  MATH  Google Scholar 

  48. Wang, X.: Semidefinite Optimization for Quantum Information. PhD Thesis, University of Technology Sydney (2018). https://opus.lib.uts.edu.au/bitstream/10453/127996/2/02whole.pdf

  49. Werner, R.F.: Quantum states with Einstein–Podolsky–Rosen correlations admitting a hidden-variable model. Phys. Rev. A 40, 4277–4281 (1989)

    Article  ADS  MATH  Google Scholar 

  50. Wang, J., Magron, V.: Exploiting term sparsity in noncommutative polynomial optimization. Comput. Optim. Appl. 80, 483–521 (2021)

    Article  MathSciNet  MATH  Google Scholar 

  51. Wan, K., Nataf, P., Mila, F.: Exact diagonalization of SU(\(N\)) Heisenberg and Affleck–Kennedy–Lieb–Tasaki chains using the full SU(\(N\)) symmetry. Phys. Rev. B 96, 115159 (2017)

    Article  ADS  Google Scholar 

  52. Zhang, F.: An update on a few permanent conjectures. Spec. Matrices 4, 305–316 (2016)

    MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Institute of Theoretical Physics, Jagiellonian University, 30-348, Kraków, Poland

    Felix Huber

  2. Faculty of Mathematics and Physics, University of Ljubljana, Ljubljana, Slovenia

    Igor Klep

  3. LAAS-CNRS, Institute of Mathematics, Toulouse, France

    Victor Magron

  4. Department of Mathematical Sciences, University of Copenhagen, Copenhagen, Denmark

    Jurij Volčič

Authors
  1. Felix Huber
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Igor Klep
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Victor Magron
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Jurij Volčič
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Jurij Volčič.

Additional information

Communicated by G. Chiribella.

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Felix Huber was supported by the FNP through TEAM-NET (POIR.04.04.00-00-17C1/18-00). Igor Klep was supported by the Slovenian Research Agency grants J1-2453, J1-8132, N1-0217 and P1-0222. Victor Magron was supported by the French Research Agency grants ANR-18-ERC2-0004-01 and ANR-19-PI3A-0004, the EU’s Horizon 2020 research and innovation programme 813211, and the PHC Proteus grant 46195TA. Jurij Volčič was supported by the National Science Foundation grant DMS-1954709, and Villum Fonden via the Villum Young Investigator grant (No. 37532).

Rights and permissions

Springer Nature or its licensor holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Huber, F., Klep, I., Magron, V. et al. Dimension-Free Entanglement Detection in Multipartite Werner States. Commun. Math. Phys. 396, 1051–1070 (2022). https://doi.org/10.1007/s00220-022-04485-9

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00220-022-04485-9

Search

Navigation